Question

Suppose that you are given a choice of receiving your scholarship in the amounts of $5,000 today and $5,000 next year, or receiving $9,000 all at once today. If the interest rate is 10%, which choice would maximize the present value of your scholarship?

Answer 1

the choice that would maximize the present value of your scholarship is receiving your scholarship in the amounts of $5,000 today and $5,000 next year.

Explanation:

Hello, I think I can help you with this

to solve this you must to find the present value in both cases, you can use this equation

`Va=(Vf)/((1+i)^(n) )`

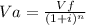
where

Va= present value

Vf=final value

n=number of periods ( in this case 1 )

i=interest rate

Step 1

option 1

$5,000 today and $5,000 next year,it would be $5000 that you receive today, and $5000 next year, let's find the presente value of the last one pay

Let

Vf=5000

i=10%=0.1

n=1,(1 year)

put the values into the equation

`Va=(Vf)/((1+i)^(n) )\\Va=(5000)/((1+0.10)^(1) )\\Va=(5000)/((1.1) )\\Va=4545.45\\`

the presente value will be

5000+4545.45= $9545.4545

you would have $9545.4545 today.

Step 2

option 2

with the second option you have $9000 today

$9545.4545 is greater than $9000

so, the choice that would maximize the present value of your scholarship is receiving your scholarship in the amounts of $5,000 today and $5,000 next year.

Have a great day