Question

Item 5 Let f(x)=2 (3) x+1 +4 . The graph of f(x) is stretched vertically by a factor of 2 to form the graph of g(x) . What is the equation of g(x) g(x) ?

Answer 1

`g(x)=4(3)^(x+1)+8`

Explanation:

Given:

The original function is given as:

`f(x)=2(3)^(x+1)+4`

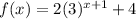
The above function is stretched vertically by a factor of 2 to form the graph of
`g(x)`.

According to the rule of function transformations, when the graph of a function is stretched in the vertical direction by a factor of 'C', where, 'C' is a number greater than 1, then the function rule is given as:

`f(x)\to Cf(x)`

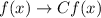
Therefore, the function is multiplied by a factor of 'C' to get the equation of the stretched function.

Here, the the value of 'C' is 2. So, the equation of
`g(x)` is given as:

`g(x)=2f(x)\\g(x)=2[2(3)^(x+1)+4]\\g(x)=4(3)^(x+1)+8`

Therefore, the equation of
`g(x)` is
`g(x)=4(3)^(x+1)+8`.