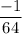Answer:
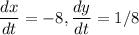
Hence, the slope ,
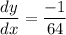
Explanation:
We need to find the slope, i.e.
 .
.
and all the functions are in terms of
 .
.
So this looks like a job for the 'chain rule', we can write:
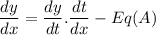
Given the functions

and
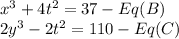
we can differentiate them both w.r.t to

first we'll derivate Eq(B) to find dx/dt
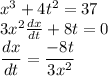
we can also rearrange Eq(B) to find x in terms of t ,
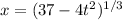 . This is done so that
. This is done so that
 is only in terms of t.
is only in terms of t.
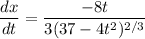
we can find the value of this derivative using t = 3, and plug that value in Eq(A).

now let's differentiate Eq(C) to find dy/dt
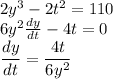
rearrange Eq(C), to find y in terms of t, that is
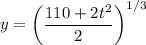 . This is done so that we can replace y in
. This is done so that we can replace y in
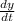 to make only in terms of t
to make only in terms of t
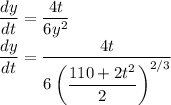
we can find the value of this derivative using t = 3, and plug that value in Eq(A).
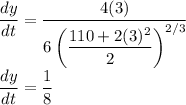
Finally we can plug all of our values in Eq(A)
but remember when plugging in the values that
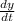 is being multiplied with
is being multiplied with
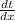 and NOT
and NOT
 , so we have to use the reciprocal!
, so we have to use the reciprocal!
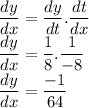
our slope is equal to