Answer:
a) W=12166.20876 J
b) U= -12166.20876 J
Step-by-step explanation:
No. of moles, n = 8.41
Change of temperature, ΔT = T1 - T2
= 395 - 279
= 116 K
For monatomic gas, γ = 5/3
γ -1 = 2 /3
Solution:
(a)
Work done,
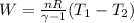
plugging values we get
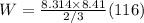
Ans: 12166.20876 J
Work done, W = + 12166.20876 J
(b)
From first law of thermodynamics, dQ = U + W
but, dQ = 0 ( adiabatic process)
Hence, U = - W
= - 12166.20876 J
Ans:
Change in internal energy, U = - 12166.20876 J