Question

The second side of a triangular deck is 6 feet longer than the shortest​ side, and the third side is 6 feet shorter than twice the length of the shortest side. If the perimeter of the deck is 68 ​feet,

what are the lengths of the three​ sides?
The shortest side of the deck is The second side of the deck is The third side of the deck is

Answer 1

The shortest side of the deck is 17 feet.

The second side of the deck is 23 feet.

The third side of the deck is 28 feet.

Explanation:

Let x represent the length of shortest side of the triangular deck.

We have been given that the second side of a triangular deck is 6 feet longer than the shortest​ side, so the length of second side would be
`x+6`.

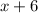We are also told that the third side is 6 feet shorter than twice the length of the shortest side, so the length of third side would be
`2x-6`.

Since the perimeter of the deck is 68 ​feet, so sum of lengths of all sides of deck would be equal to 68.

`x+x+6+2x-6=68`

Let us solve for x.

`x+x+2x+6-6=68`

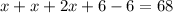
Combine like terms:

`4x=68`

Divide both sides by 4:

`(4x)/(4)=(68)/(4)`

`x=17`

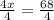
Therefore, the shortest side of the deck is 17 feet.

The length of second side would be:
`x+6\Rightarrow 17+6=23`

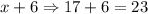
Therefore, the second side of the deck is 23 feet.

the length of third side would be:
`2x-6\Rightarrow 2\cdot 17-6=34-6=28`

Therefore, the third side of the deck is 28 feet.