Question

Beth and Pranav are selling cheesecakes for a school fundraiser. Customers can buy New York

style cheesecakes and apple cheesecakes. Beth sold 3 New York style cheesecakes and 7 apple
cheesecakes for a total of $78. Pranav sold 12 New York style cheesecakes and 12 apple
cheesecakes for a total of $168. Find the cost each of one New York style cheesecake and one
apple cheesecake.

Answer 1

Cost of one New York style cheesecake = $5

Cost of one apple cheesecake = $9

Explanation:

Let cost of one New York style cheesecake be =
`x`

Let cost of one apple cheesecake be =
`y`

Given:

3 New York stile cheesecake and 7 apple cheese cake were sold for a total of $78.

Cost of 3 New York style cheesecake be =
`3x`

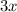
Cost of 7 apple cheesecake be =
`7y`

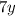
Total = $78

We have:

A)
`3x+7y=78`

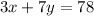
12 New York stile cheesecake and 12 apple cheese cake were sold for a total of $168.

Cost of 12 New York style cheesecake be =
`12x`

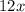
Cost of 12 apple cheesecake be =
`12y`

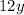
Total = $168

We have:

B)
`12x+12y=168`

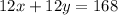
Dividing each term in equation B by 12.

`(12x)/(12)+(12y)/(12)=(168)/(12)`

`x+y=14`

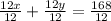
Solving for
`x` in terms of
`y`

Subtracting
`y` both sides.

`x+y-y=14-y`

`x=14-y`

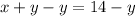
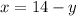
Substituting
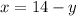`x=14-y` in equation A.

`3(14-y)+7y=78`

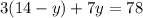
Using distribution:

`42-3y+7y=78`

`42+4y=78`

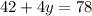
Subtracting both sides by 42.

`42+4y-42=78-42`

`4y=36`

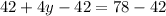
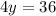
Dividing both sides by 4.

`(4y)/(4)=(36)/(4)`

∴
`y=9`

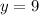
Substituting
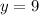`y=9` in
`x=14-y`

∴
`x=14-9=5`

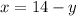
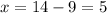
∴ Cost of one New York style cheesecake = $5

∴ Cost of one apple cheesecake = $9