Question

One number added to three times another number is 24 . Five times the first number added to three times the other number is 36 . Find the numbers

Answer 1

First number = 3

Second number = 7

Explanation:

Let one number be =
`x`

Let other number be =
`y`

"One number added to three times another number is 24" can be written mathematically as:

`x+3y=24`

Five times the first number added to three times the other number is 36 can be written mathematically as:

`5x+3y=36`

So, we have a system of equation as:

A)
`x+3y=24`

B)
`5x+3y=36`

Solving for
`x` and
`y` using elimination.

Multiplying equation A with -1 and adding it to B to eliminate
`y`

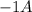`-1A` would be

`-1(x+3y=24)`

`-x-3y=-24`

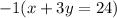
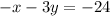
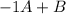`-1A+B` would be

`-x-3y=-24`

+
`5x+3y=36`

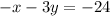
We have,

`5x-x+3y-3y=36-24`

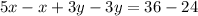
Thus
`y` is eliminated. Now, we can solve for
`x`

`4x=12`

Dividing both sides by 4.

`(4x)/(4)=(12)/(4)`

∴
`x=3`

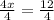
Plugging in
`x=3` in equation A to solve for
`y`

`3+3y=24`

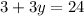
Subtracting both sides by 3.

`3+3y-3=24-3`

`3y=21`

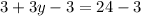
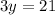
Dividing both sides by 3.

`(3y)/(3)=(21)/(3)`

∴
`y=7`

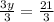
So, first number = 3

Second number = 7