Question

A heat source transfers 3000 J/sec to a metal part surface. The heated area is circular, and the heat intensity decreases as the radius increases: 75% of the heat is concentrated in a circular area that = 3.5 mm in diameter. Is the resulting power density enough to melt metal?

Answer 1

The resulting power density is enough to melt the metal.

Explanation:

Given data:

Power = P = 3000 J/sec

diameter = d = 3.5 mm

Solution:

As we Know that Area = A = π r² ---- (1)

where r is radius.

Also Radius =
`(diameter)/(2)`

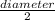
Putting the values of radius, π = 3.14 in equation 1, we get

A = 3.14 x (
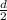`(d)/(2)`)²

A = 3.14 x (3.5/2)²

A = 9.62 mm²

As 75% of heat is concentrated in circular area then Power P becomes

P = 3000 J/sec x 75 %

As J/sec = Watt = W and 75 % = 3/4

so P = 3000 W x 3/4

P = 2250 W

As power density is represented by the formula:

Power density = PD = P/A

where P is Power and A is area.

So,

PD = P/A

Putting the values of Power and Area in above equation, we get

PD = 2250 W / 9.62 mm²

PD = 234 W/mm²

So, this power density is sufficient to melt the metal.