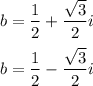Question

What are the values of ‘a’ and ‘b’?

(The ‘i’ is the imaginary number)

Answer 1

`a=\displaystyle(1)/(2)+\displaystyle(√(3))/(2)i\\\\a=\displaystyle(1)/(2)-\displaystyle(√(3))/(2)i`

`b=\displaystyle(1)/(2)+\displaystyle(√(3))/(2)i\\\\b=\displaystyle(1)/(2)-\displaystyle(√(3))/(2)i`

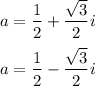
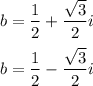
Explanation:

Recall that

`i=√(-1)`

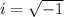
so

`i^2=-1`

we have then

`a^2+b^2=-1\\\\a+b=1`

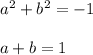
Isolating b in the second equation we get

b = 1-a

Replace this value in the first equation

`a^2+(1-a)^2=-1\Rightarrow a^2+1-2a+a^2=-1\Rightarrow\\\\\Rightarrow 2a^2-2a+2=0\Rightarrow a^2-a+1=0`

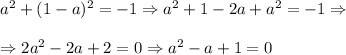
Solving the quadratic equation we get two possible solutions for a

`a=\displaystyle(1)/(2)+\displaystyle(√(3))/(2)i\\\\a=\displaystyle(1)/(2)-\displaystyle(√(3))/(2)i`

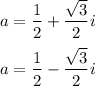
Replacing these values in b=1-a, we get two possible solutions for b

`b=\displaystyle(1)/(2)+\displaystyle(√(3))/(2)i\\\\b=\displaystyle(1)/(2)-\displaystyle(√(3))/(2)i`