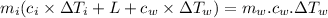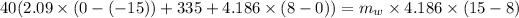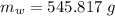Answer:
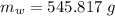
Step-by-step explanation:
Given:
- mass of ice block,
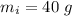
- initial temperature of ice block,

- initial temperature of water,
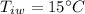
- final temperature of mixture,
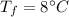
- specific heat of ice,
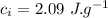
- specific heat of water,
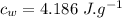
- Latent heat of fusion of water,
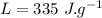
Now, assuming that there is no heat loss out of the mixture:
⇒ heat absorbed by the ice = heat rejected by the water