Question

If the volume of an orange is 288pi cm squared , then what is the radius of the fruit ?

Answer 1

Answer:
`r=6\ cm`

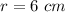
Explanation:

The volume of a sphere can be calculated with the following formula:

`V=(4)/(3)\pi r^3`

Where "V" is the volume and "r" is the radius.

In this case, you know that the volume of the orange is:

`V=288\pi`

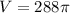
Having this value, you can substitute it into the formula:

`288\pi=(4)/(3)\pi r^3`

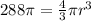
Finally, you must solve for "r" in order to calculate the radius ot the orange.

This is:

`3(288\pi)=4\pi r^3\\\\\sqrt[3]{(3(288\pi))/(4\pi ) }=r\\\\r=6\ cm`