Answer:
x = −1, 1
Explanation:
Vertical Asymptotes
A vertical asymptote of the graph of a given function f(x) is the line x=a, such that one of of these statements is fulfilled
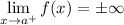
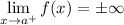
If f(x) is a rational expression, we must find all the values of x who make the denominator equal to zero
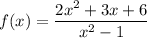
We set the denominator to zero
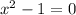
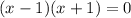

Those are the vertical asymptotes of f