Question

ASAP can somebody answer this? SCREEN SHOT OF QUESTION ATTACHED

Answer 1

A.
`(-\infty, -4]\ or\ (2,\infty)`

Explanation:

Given:

The inequality is given as:

`x\leq-4\ or\ x>2`

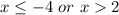
Now, consider the first inequality

`x\leq-4`

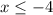
Here, 'x' is less than or equal to -4. The values that are less than -4 are -5, -6, -7... so on. The inequality used is 'less than or equal to'. This means that -4 is included in the solution. So, we use a square bracket (closed interval) at the other end.

The inequality in notation form is thus,
`(-\infty,-4]`

Now, consider the other inequality
`x>2`

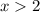
The values of 'x' are greater than 2. The values that are greater than 2 are 3, 4, 5... and so on. Also, 2 is not included in the solution. So, we use open interval on either side.

Therefore,
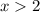`x>2` in interval notation form is
`(2,\infty)`

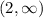
There is a conjunction 'or' used in the inequality. Therefore, the answer is:

A.
`(-\infty, -4]\ or\ (2,\infty)`

The graph on the number line is shown below.