Answer:
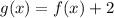
 is shifted 2 units upwards to become
is shifted 2 units upwards to become

Explanation:
Given functions:
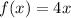
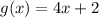
On comparing the functions we find out that:
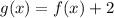
The translation rule for this is given as:

If
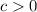 the function shifts
the function shifts
 units to the up.
units to the up.
If
 the function shifts
the function shifts
 units to the down.
units to the down.
Thus we can see the unit shift from
 to
to
 is 2 units upwards as 2 is being added to function
is 2 units upwards as 2 is being added to function
