To solve the problem it is necessary to find the total power generated by each of the panels and then through a proportional relationship find the number of total panels.
There is an efficiency of 18% of which the area of
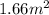 receives a surface radiation of
receives a surface radiation of
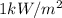
Therefore the power is subject to

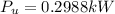
Each panel will have the capacity to produce 0.2998kW
The number of panels required is determined by the total power given by each of the panels, that is
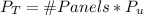
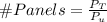
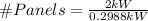
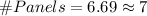
Therefore the number of panels required are 7