Question

The vapor pressure of dichloromethane, CH2Cl2, at 0 ∘C is 134 mmHg. The normal boiling point of dichloromethane is 40. ∘C. Calculate its molar heat of vaporization.

Answer 1

The molar heat of vaporization can be calculated using the Clausius-Clapeyron equation. To calculate it, we need the initial temperature, the initial vapor pressure, the final temperature, and the final vapor pressure. By rearranging the equation and plugging in the given values, we can find the molar heat of vaporization.

Step-by-step explanation:

The molar heat of vaporization can be calculated using the Clausius-Clapeyron equation:

ln(P₁/P₂) = (ΔHvap/R)((1/T₂) - (1/T₁))

Where:

• P₁ is the vapor pressure at T₁
• P₂ is the vapor pressure at T₂
• ΔHvap is the enthalpy of vaporization
• R is the gas constant, which is 8.314 J/mol·K
• T₁ is the initial temperature (in Kelvin)
• T₂ is the final temperature (in Kelvin)

In this case, T₁ = 273 K (0 °C + 273) and P₁ = 134 mmHg. We need to find T₂. Plugging in the values:

ln(134/P₂) = (-ΔHvap/8.314)((1/T₂) - (1/273))

Rearranging the equation to solve for T₂:

T₂ = 1 / ((ΔHvap/8.314) / ln(134/P₂) + (1/273))

Now we can substitute the given values: ΔHvap = ? and P₂ = 760 mmHg (the vapor pressure at the boiling point).

Answer 2

Answer: The molar heat of vaporization is 30.6 kJ/mol

Step-by-step explanation:

To calculate the molar heat of vaporization, we use the equation given by Clausius-Clapeyron, which is:

`\ln ((P_1)/(P_2))=-(\Delta H_(vap))/(R)\left((1)/(T_1)-(1)/(T_2)\right )`

Where,

`P_1` = vapor pressure at temperature
`T_1` = 134 mmHg

`P_2` = vapor pressure at temperature
`T_2` (atmospheric pressure) = 760 mmHg

`\Delta H_(vap)` = molar heat of vaporization

R = gas constant = 8.314 J/mol.K

`T_1` = temperature of dichloromethane =
`0^oC=[273+0]K=273K`

`T_2` = normal boiling point of dichloromethane =
`40^oC=[273+40]K=313K`

Putting values in above equation, we get:

`\ln (136)/(760)=-(\Delta H_(vap))/(8.314J/mol.K)\left((1)/(273)-(1)/(313)\right)\\\\\Delta H_(vap)=30559.97J/mol=30.55kJ/mol\approx 30.6kJ/mol`

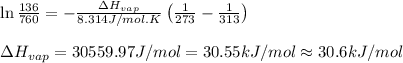
Hence, the molar heat of vaporization is 30.6 kJ/mol