Question

What is the distance between the points A(2, -1) and B(-4, 2)

A). 2
B).
`\sqrt[3]{5}`
C).
`\sqrt[5]{3}`
D).4

Answer 1

The distance between two points A(2, -1) and B(-4, 2) is
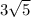`3 √(5)` units

Solution:

Given that two points are A(2, -1) and B(-4, 2)

To find: distance between two points

The distance between two points
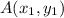`A(x_1, y_1)` and
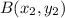`B(x_2, y_2)` is given as:

`Distance(A, B) = d = √((x_2 - x_1)^2+ (y_2 - y_1)^2)`

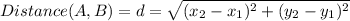
Here in this problem,

`x_1 = 2 ; y_1 = -1 ; x_2 = -4 ; y_2 = 2`

Substituting the values in given formula, we get

`d = √((-4-2)^2+(2-(-1))^2)\\\\d = √((-6)^2 + (3)^2)\\\\d = √(36 + 9)\\\\d = √(45)`

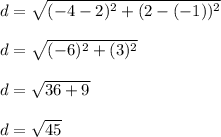
On simplification we get,

`d = √(45) = √(3 * 3 * 5)\\\\d = 3√(5)`

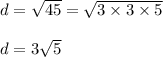
Thus the distance between two points A(2, -1) and B(-4, 2) is
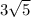`3 √(5)` units