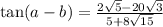Answer:
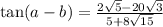
Explanation:
I'm going to use the following identity to help with the difference inside the tangent function there:
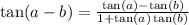
Let
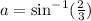 .
.
With some restriction on
 this means:
this means:
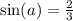
We need to find
 .
.
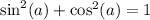 is a Pythagorean Identity I will use to find the cosine value and then I will use that the tangent function is the ratio of sine to cosine.
is a Pythagorean Identity I will use to find the cosine value and then I will use that the tangent function is the ratio of sine to cosine.
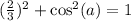
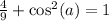
Subtract 4/9 on both sides:
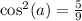
Take the square root of both sides:
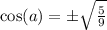
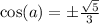
The cosine value is positive because
 is a number between
is a number between
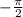 and
and
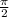 because that is the restriction on sine inverse.
because that is the restriction on sine inverse.
So we have
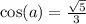 .
.
This means that
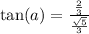 .
.
Multiplying numerator and denominator by 3 gives us:

Rationalizing the denominator by multiplying top and bottom by square root of 5 gives us:
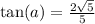
Let's continue on to letting
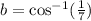 .
.
Let's go ahead and say what the restrictions on
 are.
are.
 is a number in between 0 and
is a number in between 0 and
 .
.
So anyways
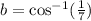 implies
implies
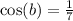 .
.
Let's use the Pythagorean Identity again I mentioned from before to find the sine value of
 .
.

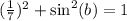

Subtract 1/49 on both sides:
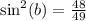
Take the square root of both sides:

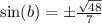
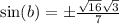

So since
 is a number between
is a number between
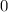 and
and
 , then sine of this value is positive.
, then sine of this value is positive.
This implies:
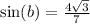
So
 .
.
Multiplying both top and bottom by 7 gives:
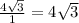 .
.
Let's put everything back into the first mentioned identity.
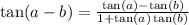
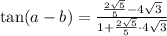
Let's clear the mini-fractions by multiply top and bottom by the least common multiple of the denominators of these mini-fractions. That is, we are multiplying top and bottom by 5: