Answer:
The equation of the line perpendicular to the line y = 3 x - 4 is x + 3 y + 1 = 0
Explanation:
Given line equation as :
y = 3 x - 4
Now a line which is perpendicular to given line passes through point p ( 3 , - 2 )
The standard equation of line is given as
y = m x + c
where m is the slope of the line
Now, for line y = 3 x - 4
So By comparing the line, the slope of this line = m = 3
Now, when two lines are perpendicular then
The product of the slope of lines = - 1
Let the slope of other line = M
So, from property
m × M = - 1
∴ M =

Or, M =
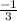
∴ M =
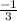
Now, The line having slope M =
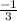 , is passing through point p ( 3 , - 2 )
, is passing through point p ( 3 , - 2 )
From standard equation of line
I.e y = m x + c
As the line y = m x + c passes through point ( 3 , - 2 ) having slope M =
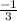
So, satisfying the points and slope in equation y = M x + c
I.e - 2 =
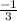 ( 3 ) + c
( 3 ) + c
Or, - 2 =
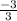 ( 3 ) + c
( 3 ) + c
Or, - 2 = - 1 + c
∴ c = - 2 + 1
i.e c = - 1
So The equation of line can be written as
y =
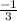 x - 1
x - 1
or, 3 y = - x - 1
or, x + 3 y + 1 = 0
So equation of new line is x + 3 y + 1 = 0
Hence The equation of the line perpendicular to the line y = 3 x - 4 is x + 3 y + 1 = 0 . Answer