Question

Write the phrase as an expression. Then evaluate when y=20.

the quotient of 40 and the difference of a number y and 16.

Answer 1

`Q=(40)/(y-16)`

`Q=10`

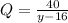
Explanation:

Let the quotient be represented by 'Q'.

Given:

The difference of a number 'y' and 16 is
`y-16`

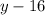
Quotient is the answer that we get on dividing two terms. Here, the first term is 40 and the second term is
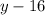`y-16`. So, we divide both these terms to get an expression for 'Q'.

The quotient of 40 and
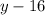`y-16` is given as:

`Q=(40)/(y-16)`

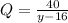
Now, we need to find the quotient when
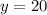`y=20`. Plug in 20 for 'y' in the above expression and evaluate the quotient 'Q'. This gives,

`Q=(40)/(20-16)\\Q=(40)/(4)=10`

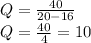
Therefore, the quotient is 10, when the value of 'y' is 20.