Question

A horizontal uniform bar of mass 2.7 kg and length 3.0 m is hung horizontally on two vertical strings. String 1 is attached to the end of the bar, and string 2 is attached a distance 0.65 m from the other end. A monkey of mass 1.35 kg walks from one end of the bar to the other. Find the tension T1 in string 1 at the moment that the monkey is halfway between the ends of the bar

Answer 1

14.36 N

Step-by-step explanation:

`T_(1)` = Tension in string 1

`T_(2)` = Tension in string 2

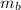`m_b` = mass of the bar = 2.7 kg

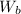`W_b` = weight of the bar

weight of the bar is given as

`W_b = m_(b) g = (2.7) (9.8) = 26.46`N

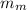`m_m` = mass of the bar = 1.35 kg

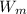`W_m` = weight of the monkey

weight of the monkey is given as

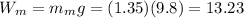`W_m = m_(m) g = (1.35) (9.8) = 13.23`N

Using equilibrium of torque about left end

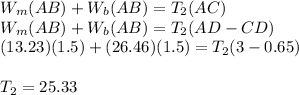`W_(m) (AB) + W_(b) (AB) = T_(2) (AC)\\W_(m) (AB) + W_(b) (AB) = T_(2) (AD - CD)\\(13.23) (1.5) + (26.46)(1.5) = T_(2) (3 - 0.65)\\\\T_(2) = 25.33` N

Using equilibrium of force in vertical direction

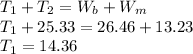`T_(1) + T_(2) = W_(b) + W_(m)\\T_(1) + 25.33 = 26.46 + 13.23\\T_(1) = 14.36` N