Question

Head movement evaluations are important because disabled individuals may be able to operate communications aids using head motion. A paper reported the accompanying data on neck rotation (in degrees) for 14 subjects both in the clockwise direction (CL) and in the counterclockwise direction (CO). For purposes of this exercise, you can assume that the 14 subjects are representative of the population of adult Americans.

Subject: 1 2 3 4 5 6 7 8 9 10 11 12 13 14
CL: 57.8 35.7 54.5 56.8 51.1 70.8 77.3 51.6 54.7 63.6 59.2 59.2 55.8 38.5
CO: 44.7 52.1 60.2 52.7 47.2 65.6 71.4 48.8 53.1 66.3 59.8 47.5 64.5 34.4
Based on these data, is it reasonable to conclude that mean neck rotation is greater in the clockwise direction than in the counterclockwise direction? Carry out a hypothesis test using a significance level of 0.01. (Use a statistical computer package to calculate the P-value. Use μCL − μCO. Round your test statistic to two decimal places and your P-value to three decimal places.)

t =
df =
P-value =

Answer 1

`t=(\bar d -0)/((s_d)/(√(n)))=(1.307 -0)/((7.798)/(√(14)))=0.6271`

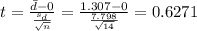
df=n-1=14-1=13

`p_v =P(t_((13))>0.6271) =0.2707`

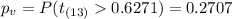
Explanation:

A paired t-test is used to compare two population means where you have two samples in which observations in one sample can be paired with observations in the other sample. For example if we have Before-and-after observations (This problem) we can use it.

Let put some notation

x=test value CL , y = test value CO

x: 57.8 35.7 54.5 56.8 51.1 70.8 77.3 51.6 54.7 63.6 59.2 59.2 55.8 38.5

y: 44.7 52.1 60.2 52.7 47.2 65.6 71.4 48.8 53.1 66.3 59.8 47.5 64.5 34.4

The system of hypothesis for this case are:

Null hypothesis:
`\mu_x- \mu_y \leq 0`

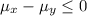
Alternative hypothesis:
`\mu_x -\mu_y >0`

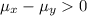
The first step is calculate the difference
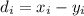`d_i=x_i-y_i` and we obtain this:

d: 13.1, -16.4,-5.7,4.1,3.9,5.2,5.9,2.8,1.6,-2.7,-0.6,11.7,-8.7,4.1

The second step is calculate the mean difference

`\bar d= (\sum_(i=1)^n d_i)/(n)= (29)/(8)=1.307`

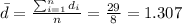
The third step would be calculate the standard deviation for the differences, and we got:

`s_d =(\sum_(i=1)^n (d_i -\bar d)^2)/(n-1) =7.798`

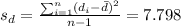
The 4 step is calculate the statistic given by :

`t=(\bar d -0)/((s_d)/(√(n)))=(1.307 -0)/((7.798)/(√(14)))=0.6271`

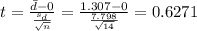
The next step is calculate the degrees of freedom given by:

`df=n-1=14-1=13`

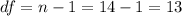
Now we can calculate the p value, since we have a right tailed test the p value is given by:

`p_v =P(t_((13))>0.6271) =0.2707`

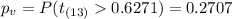
So the p value is higher than the significance level provided of 0.01, so then we can conclude that we FAIL to reject the null hypothesis that the difference mean between after and before is higher or equal than 0. So we can't conclude that the mean neck rotation is greater in the clockwise direction than in the counterclockwise direction at 1% of significance.