Question

A storm blows wind across the top of a roof at a speed of v = 26.3 m/s. Assume the air on the lower side of the roof (inside) is at rest and the roof is flat and has an area A = 38.9 m². The density of air is 1.28 kg/m³.1) What is the pressure differential across the top and bottom of the roof? 2) What is the net lift force on the roof due to the storm?

Answer 1

1)

442.7 Pa

2)

17221 N

Step-by-step explanation:

1)

`\rho` = density of air = 1.28 kg/m

`v` = speed of wind at the top of rood = 26.3 m/s

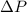`\Delta P` = pressure difference across the top and bottom of the roof

Pressure difference across the top and bottom of the roof is given as

`\Delta P = (0.5) \rho v^(2)`

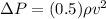
inserting the values

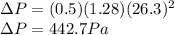
`\Delta P = (0.5) (1.28) (26.3)^(2)\\\Delta P = 442.7 Pa`

2)

`F` = lift force

`A` = area of the roof = 38.9 m²

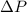`\Delta P` = pressure difference across the top and bottom of the roof = 442.7 Pa

we know that

`F = P A\\F = (442.7) (38.9)\\F = 17221 N`