Answer:
Approximately
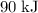 .
.
Step-by-step explanation:
Cathode is where reduction takes place and anode is where oxidation takes place. The potential of a electrochemical reaction (
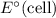 ) is equal to
) is equal to
 .
.
There are two half-reactions in this question.
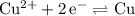 and
and
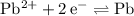 . Either could be the cathode (while the other acts as the anode.) However, for the reaction to be spontaneous, the value of
. Either could be the cathode (while the other acts as the anode.) However, for the reaction to be spontaneous, the value of
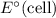 should be positive.
should be positive.
In this case,
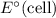 is positive only if
is positive only if
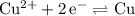 is the reaction takes place at the cathode. The net reaction would be
is the reaction takes place at the cathode. The net reaction would be
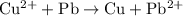 .
.
Its cell potential would be equal to
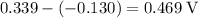 .
.
The maximum amount of electrical energy possible (under standard conditions) is equal to the free energy of this reaction:
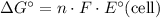 ,
,
where
 is the number moles of electrons transferred for each mole of the reaction. In this case the value of
is the number moles of electrons transferred for each mole of the reaction. In this case the value of
 is
is
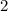 as in the half-reactions.
as in the half-reactions.
 is Faraday's Constant (approximately
is Faraday's Constant (approximately
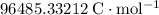 .)
.)
 .
.