Answer: 1.30 in.
Explanation:
Let x denotes the height of cabinets.
We are given that ,
In a sample of 66 cabinets, the average height was found to be 36.5 in. with a variance of 1.7.
i.e. sample mean =
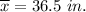
Sample variance =
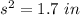 .
.
Since standard deviation is the square root of variance.
i.e. Sample standard deviation :
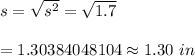
Since the sample standard deviation(s) is the best point-estimate of the population standard deviation
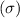 .
.
Then, a point estimate for the population standard deviation
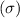 of the height of the cabinets.= 1.30 in.
of the height of the cabinets.= 1.30 in.