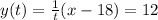Answer:
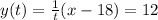
Explanation:
The tangent of a curve is given by the following first degree function:

In which
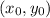 is a function in which the curve pass through and m is the slope, given by the following formula:
is a function in which the curve pass through and m is the slope, given by the following formula:
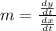
In this problem, we have that:
 .
.
So:
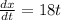
And
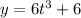
So:
 .
.
Which means that

The curve passes through (18,12). This means that
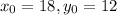 .
.
So the equation is: