Answer:
a) 45g of ice will melt, final temperature of mixture will be 90.8 °C
b) 10.4g of ice will melt, final temperature of mixture will be 0 °C
Step-by-step explanation:
The energy lost by the steam is the sum of the latent heat of vaporization of steam and the energy lost in bringing the temperature of the mixture down to the equilibrium temperature. This is equal to the energy gained by the ice, which is the sum of the latent heat of fusion and the energy gained in raising the temperature of the ice to the equilibrium temperature.
Part a)
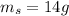 ,
,
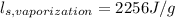 ,
,
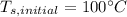
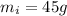 ,
,
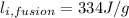 ,
,

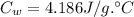
Step 1: Determine energy needed to melt ice
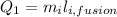
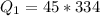

Step 2: Determine energy released during condensation of steam
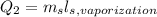
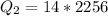

Step 3: Determine temperature of increase of ice water
Energy released in condensation of steam exceeds energy required for melting of ice. Excess energy will raise the temperature of the ice water.
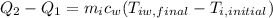

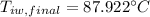
Step 4: Determine average temperature of water
The temperature of water from condensation of steam
 will be 100 °C
will be 100 °C
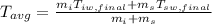
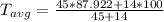
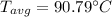
Part b)
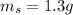 ,
,
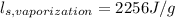 ,
,
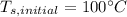
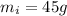 ,
,
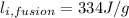 ,
,

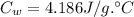
Step 1: Determine energy released during condensation of steam

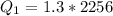

Step 2: Determine energy released during cooling of steam water to 0°C
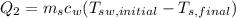
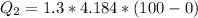
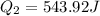
Step 3: Determine mass of ice melted
Energy released by condensation of steam and cooling of water is used to melt ice


Entire mixture temperature is at 0°C as steam condenses and cools to 0°C, ice melts and remains at 0°C.