Answer:
-8
Explanation:
Given:
The expression is given as:
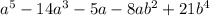
Rearranging the terms in standard form with the largest exponent as the first term and smallest exponent as the last term. This gives,
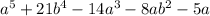
Now, the fourth term is
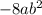 .
.
The coefficient is the constant value of the term. The given polynomial has two variables 'a' and 'b'. The constant value in the fourth term is -8.
So, the coefficient of the fourth term of the given expression is -8.