Question

The Sun orbits the center of the Milky Way galaxy once each 2.60 × 108 years, with a roughly circular orbit averaging 3.00 × 104 light years in radius. (A light year is the distance traveled by light in 1 y.)

Calculate the centripetal acceleration of the Sun in its galactic orbit. Does your result support the contention that a nearly inertial frame of reference can be located at the Sun?

Answer 1

To solve this problem it is necessary to apply the kinematic equations of linear and angular motion, as well as the given definitions of the period.

Centripetal acceleration can be found through the relationship

`a_c = (v^2)/(R)`

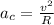
Where

v = Tangential Velocity

R = Radius

At the same time linear velocity can be expressed in terms of angular velocity as

`v = R\omega`

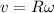
Where,

R = Radius

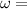`\omega =` Angular Velocity

PART A) From this point on, we can use the values used for the period given in the exercise because the angular velocity by definition is described as

`\omega = (2\pi)/(T)`

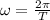
T = Period

So replacing we have to

`\omega = (2\pi)/(2.6*10^8years)\\\omega = 2.4166*10^(-8)rad/years\\\omega = 2.4166*10^(-8)rad/years((1years)/(365days))((1day)/(86400s))\\\omega = 7.663*10^(-16)rad/s`

Since
`1 Light year = 9.48*10^(15)m`

Then the radius in meters would be

`R = (3*10^4ly)((9.48*10^(15)m)/(1ly))`

`R = 2.844*10^(20)m`

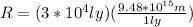
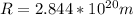
Then the centripetal acceleration would be

`a_c = (v^2)/(R)\\a_c = ((R\omega)^2)/(R)\\a_c = R\omega^2 \\a_c = 2.844*10^(20)(7.663*10^(-16))^2\\a_c = 1.67*10^(-10)m/s^2`

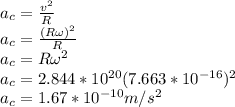
From the result obtained, considering that it is an unimaginably low value of an order of less than
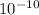`10^(-10)` it is possible to conclude that it supports the assertion on the inertial reference frame.