Question

Three fire hoses are connected to a fire hydrant. Each hose has a radius of 0.025 m. Water enters the hydrant through an underground pipe of radius 0.075 m. In this pipe the water has a speed of 3.1 m/s. (a) How many kilograms of water are poured onto a fire in one hour by all three hoses? kg (b) Find the water speed in each hose. m/s

Answer 1

given,

radius of each hose = 0.025 m

water enters hydrant at radius = 0.075 m

speed of water in the pipe = 3.1 m/s

a) mass of water per hours through the house can be calculated

`(dM)/(dt) = \rho AV`

`(dM)/(dt) = \pi r^2 \rho V`

`(dM)/(dt) = \pi * 0.075^2* 1000 * 3.1`

`(dM)/(dt) =54.78\ Kg/s`

`(dM)/(dt) =54.78* 3600\ Kg/h`

`(dM)/(dt) =1.97* 10^5\ Kg/h`

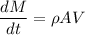
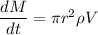
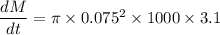
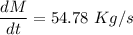
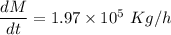
b) Using continuity equation

`A_1V_1 = A_2V_2`

`\pi * 0.075^2* 3.1 = 3 * \pi * 0.025^2\ V_2`

v₂ = 9.3 m/s

speed of water through each hose.