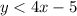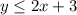Question

The shaded region in the graph represents the solutions of this system of linear inequalities:

y ___ 4x + ____

y ≤ ___ x + ____

Answer 1

`y<4x-5`

`y\leq 2x+3`

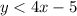
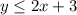
Explanation:

step 1

Find the equation of the solid line

From the graph take the points (0,3) and (4,11)

Find the slope

`m=(11-3)/(4-0)\\m=8/4=2`

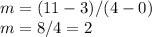
The equation of the solid line in slope intercept form is equal to

`y=mx+b`

we have

`m=2`

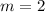
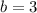`b=3` ----> the y-intercept is the point (0,3)

substitute

`y=2x+3`

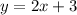
therefore

The inequality is

`y\leq 2x+3`

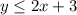
step 2

Find the equation of the dashed line

The slope is given

`m=4`

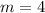
From the graph take the y-intercept (0,-5)

The equation of the solid line in slope intercept form is equal to

`y=mx+b`

we have

`m=4`

`b=-5`

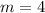
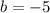
substitute

`y=4x-5`

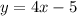
therefore

The inequality is

`y<4x-5`

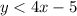
because the shaded region is below the dashed line

therefore

The system of inequalities is

`y<4x-5`

`y\leq 2x+3`