Question

Wild fruit flies have red eyes. A recessive mutation produces white-eyed individuals. A researcher wants to assess the proportion of heterozygous individuals. A heterozygous red-eyed fly can be identified through its off-spring. When crossed with a white-eyed fly it will have a mixed progeny.A random sample of 100 red-eyed fruit flies was taken. Each was crossed with a whiteeyed fly. Of the sample flies, 11 were shown to be heterozygous because they produced mixed progeny.a) Check this data for the conditions necessary for the calculation of a large-sample confidence interval. Does it comply OR should you use the plus-four interval only?b) Determine a 95% confidence interval for the proportionc) Also use a test of significance at 5% to test the hypothesis that the proportion of heterozygous red-eyed flies is different from 10 %?d) Compare the answer from this test at 5% in c) to that from the 95% confidence interval in b). Would you necessarily expect the same answer?

Answer 1

a)
`np=100*0.11=11>10`

`n(1-p)=100*(1-0.11)=89>10`

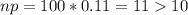
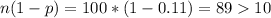
The conditions are satisfied to assume that the proportion is distributed normally so we can calculate the confidence interval and conduct the test hypothesis.

b) The 95% confidence interval would be given (0.0487;0.171).

c)
`p_v =2*P(z>0.33)=0.741`

If we compare the p value and the significance level given
`\alpha=0.05` we have
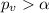`p_v>\alpha` so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and the true population proportion is not significantly different from 0.1.

d) On both cases we got the same conclusion the population proportion is not significantly different from 0.1 at 5% of singificance.

Explanation:

Data given and notation n

n=100 represent the random sample taken

X=11 represent the number with heterozygous because they produced mixed progeny.

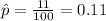`\hat p=(11)/(100)=0.11` estimated proportion with with heterozygous because they produced mixed progeny.

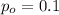`p_o=0.1` is the value that we want to test

`\alpha=0.05` represent the significance level

Confidence=95% or 0.95

z would represent the statistic (variable of interest)

`p_v` represent the p value (variable of interest)]

a) Check this data for the conditions necessary for the calculation of a large-sample confidence interval. Does it comply OR should you use the plus-four interval only?

`np=100*0.11=11>10`

`n(1-p)=100*(1-0.11)=89>10`

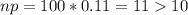
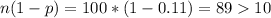
The conditions are satisfied to assume that the proportion is distributed normally so we can calculate the confidence interval and conduct the test hypothesis.

b) Determine a 95% confidence interval for the proportion

The confidence interval would be given by this formula

`\hat p \pm z_(\alpha/2) \sqrt{(\hat p(1-\hat p))/(n)}`

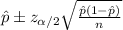
For the 95% confidence interval the value of
`\alpha=1-0.95=0.05` and
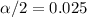`\alpha/2=0.025`, with that value we can find the quantile required for the interval in the normal standard distribution.

`z_(\alpha/2)=1.96`

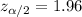
And replacing into the confidence interval formula we got:

`0.11 - 1.96 \sqrt{(0.11(1-0.11))/(100)}=0.0487`

`0.11 + 1.96 \sqrt{(0.11(1-0.11))/(100)}=0.171`

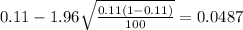
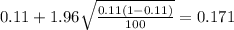
And the 95% confidence interval would be given (0.0487;0.171). And we see that the interval contains 0.1.

c) Also use a test of significance at 5% to test the hypothesis that the proportion of heterozygous red-eyed flies is different from 10 %?

Null hypothesis:
`p=0.1`

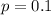
Alternative hypothesis:
`p \\eq 0.1`

When we conduct a proportion test we need to use the z statistic, and the is given by:

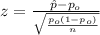`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Calculate the statistic

Since we have all the info requires we can replace in formula (1) like this:

`z=\frac{0.11 -0.1}{\sqrt{(0.1(1-0.1))/(100)}}=0.33`

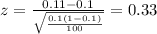
Statistical decision

It's important to refresh the p value method or p value approach . "This method is about determining "likely" or "unlikely" by determining the probability assuming the null hypothesis were true of observing a more extreme test statistic in the direction of the alternative hypothesis than the one observed". Or in other words is just a method to have an statistical decision to fail to reject or reject the null hypothesis.

The significance level provided
`\alpha=0.05`.

Since is a bilateral test the p value would be:

`p_v =2*P(z>0.33)=0.741`

If we compare the p value and the significance level given
`\alpha=0.05` we have
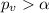`p_v>\alpha` so we can conclude that we have enough evidence to FAIL to reject the null hypothesis, and the true population proportion is not significantly different from 0.1.

d) Compare the answer from this test at 5% in c) to that from the 95% confidence interval in b). Would you necessarily expect the same answer?

On both cases we got the same conclusion the population proportion is not significantly different from 0.1 at 5% of singificance.