Answer:
The roots of the equation for the given quadratic equation is
 .
.
Explanation:
Given quadratic equation as :
2 x² + 8 x + 5 = 0
The roots of the quadratic equation ax² + bx + c = 0 as can be find as :
x =
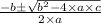
x =
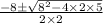
or, x =
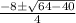
Or, x =
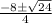
Or, x =
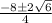
∴ x =

Hence The roots of the equation for the given quadratic equation is
 . Answer
. Answer