Question

Let z=6-7i and z^2=a+bi. What are the values of a and b ?

Answer 1

The values are

a = -13

b = -84

Explanation:

Given:

z=6-7i and

`z^(2)=a + bi`

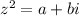
To Find:

a = ?

b = ?

Solution:

Z is Complex Number consist of Real part and Imaginary part

We have

`z=6-7i\\\textrm{squaring on both the side we get}\\z^(2)=(6-7i)^(2)`

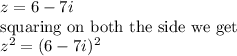
Using the identity
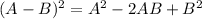`(A-B)^(2) =A^(2)-2AB+ B^(2)` we get

`z^(2) =6^(2)-2* 6* 7i+ (7i)^(2)\\z^(2) =36-84i+49i^(2)\\`

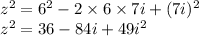
i² = -1

∴
`z^(2) =36-84i+49(-1)\\z^(2) =36-49-84i\\z^(2) =-13-84i`

Now on comparing with
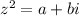`z^(2)=a + bi` equation we get

a = -13

b = -84