Question

Why is the product of two rational numbers always rational?

Select from the drop-down menus to correctly complete the proof.

Answer 1

The product of two rational numbers is always rational

Explanation:

• DEFINITION: a number is said to be rational if and only if it is expressed in p/q form i.e, as a fraction(p/q) where, p,q are integers and
  `q\\eq 0.`

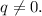
• now, let a/b and c/d be two rational numbers.

• the product of them : ac/bd.

• FACT : if we multiply 2 integers, then the product will be an integer.

• so, ac and bd are both integers for sure and bd is not zero because none of b or d is zero.

• therefore, as ac/bd satisfy the definition of a rational number, it is a rational number.

• hence, we can now generalize that, The product of two rational numbers is always rational.