Question

Help me find the area.↓

Answer 1

46 sq, units.

Explanation:

Let the quadrilateral has vertices A(-4,-2), B(2,-4), C(6,2) and D(-6,2).

Let us draw a diagonal AC connecting vertices A and C.

Now, Area of ABCD = Area of Δ ABC + Area of Δ ACD

Now, area of Δ ABC is given by

`(1)/(2) |- 4(- 4 - 2) + 2(2 - (- 2)) + 6( -2 - (-4))| = 22` sq. units.

Again, the area of Δ ACD is given by

`(1)/(2) |- 4 (2 - 2) + 6(2 - (- 2)) - 6(- 2 - 2) | = 24` sq. unirs.

Therefore the are of the quadrilateral is = 22 + 24 = 46 sq. units. (Answer)

If (
`x_(1),y_(1)`), (
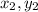`x_(2),y_(2)`), and (
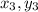`x_(3),y_(3)`) are the three vertices of a triangle then the area of the triangle is given by

`(1)/(2) |x_(1)(y_(2) - y_(3)) + x_(2)(y_(3) - y_(1)) + x_(3)(y_(1) - y_(2)) |` sq. units.