Question

Given the following information about a hypothesis test of the difference between two means based on independent random samples, what is the standard deviation of the difference between the two means? Assume that the samples are obtained from normally distributed populations having equal variances.H0: ?A = ?B, and H1: ?A > ?B X ¯ 1 = 12, X ¯ 2 = 9, s1= 5, s2 = 3, n1 =13, n2 =10.A. 1.792B. 1.679C. 2.823D. 3.210E. 1.478

Answer 1

Answer: B. 1.679

Explanation:

The standard deviation for the difference between two mean is given by :-

`SD_{\overline{x}_1-\overline{x}_2}=\sqrt{(s_1^2)/(n_1)+(s_2^2)/(n_2)}`

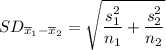
, where
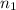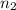`n_1` = sample size from population 1.

`n_2` = sample size from population 2.

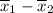`\overline{x}_1-\overline{x}_2` = sample mean differnce.

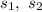`s_1,\ s_2` = sample standard deviations .

Given :
`{\overline{x}_1=12,\ \ \overline{x}_2=9`

`s_1=5,\ \ s_2=3`

`n_1=13,\ n_2=10`

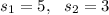
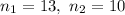
Then, the standard deviation of the difference between the two means will be :

`SD_{\overline{x}_1-\overline{x}_2}=\sqrt{((5)^2)/(13)+((3)^2)/(10)}`

`=\sqrt{(25)/(13)+(9)/(10)}\\\\=\sqrt{(367)/(130)}=1.68020145312\approx1.680`

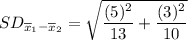
The nearest option : B. 1.679

Hence, the standard deviation of the difference between the two means = 1.679