Answer:
The volume displaced is 6.2 cubic meters.
Step-by-step explanation:
This question is incomplete.
However, we can find the volume displaced using the given mass and the density of brass.
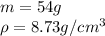
So, to find the volume, we use the definition of density, which is
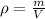
Where
 is density,
is density,
 is mass and
is mass and
 is volume.
is volume.
Replacing all values, we have

Therefore, the volume displaced is 6.2 cubic meters.