Question

Use the given information to find the number of degrees of​ freedom, the critical values chi Subscript Upper L Superscript 2 and chi Subscript Upper R Superscript 2​, and the confidence interval estimate of sigma. It is reasonable to assume that a simple random sample has been selected from a population with a normal distribution. Nicotine in menthol cigarettes 98​% ​confidence; nequals28​, sequals0.22 mg.

Answer 1

Chi-Square value for lower bond: X²
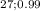`_(27;0.99)`= 46963

Chi-Square value for upper bond: X²
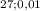`_(27;0,01)`= 12.878

Confidence interval: [0.0278;0.1001]

Explanation:

Hello!

You need to make a 98% Confidence interval for the population variance of a single sample. To construct it you have to use a Chi-Square statistic:

X²= (n-1)S² ~X²
`_(n-1)`

σ²

The formula for the interval is:

Lower bond:

(n-1)S² = 27*0.0484 = 1.3068 = 0.0278

X²
`_(n-1;1-α/2)` X²
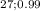`_(27;0.99)` 46.963

Upper bond:

(n-1)S² = 2*0.0484 = 1.3068 = 0.1001

X²
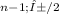`_(n-1;α/2)` X²
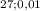`_(27;0,01)` 12.878

n=28

S= 0.22

S²=0.0484

With a 98% confidence level, you'd expect the true value of the nicotine variance in menthol cigarettes is contained by the interval [0.0278;0.1001]

I hope it helps!