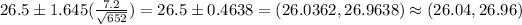Question

An NHANES report gives data for 652 women aged 20 – 29 20–29 years. The BMI of these 652 652 women was ¯ x = 26.5 x¯= 26.5 . On the basis of this sample, we want to estimate the BMI μ μ in the population of all 20.6 20.6 million women in this age group. We treated these data as an SRS from a Normally distributed population with standard deviation σ = 7.2 σ=7.2 . Give three confidence intervals for the mean BMI μ in this population, using 90%, 95%, and 99% confidence. Enter the lower and upper bound for the 90% confidence interval.

Answer 1

Lower bound of 90% confidence interval: 26.04

Upper bound of 90% confidence interval: 26.96

Explanation:

We are given the following in the question:

Sample mean,
`\bar{x}` = 26.5

Sample size, n = 652

Population standard deviation, σ = 7.2

90% Confidence interval:

`\mu \pm z_(critical)(\sigma)/(√(n))`

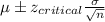
Putting the values, we get,

`z_(critical)\text{ at}~\alpha_(0.05) = 1.645`

`26.5 \pm 1.645((7.2)/(√(652)) ) = 26.5 \pm 0.4638 =(26.0362,26.9638) \approx (26.04,26.96)`