Question

A binary geothermal power plant uses geothermal water at 160°C as the heat source. The cycle operates on the simple Rankine cycle with isobutane as the working fluid. Heat is transferred to the cycle by a heat exchanger in which geothermal liquid water enters at 160EC at a rate of 555.9 kg/s and leaves at 90°C. Isobutane enters the turbine at 3.25 MPa and 147°C at a rate of 305.6 kg/s, and leaves at 79.5°C and 410 kPa. Isobutane is condensed in an air-cooled condenser and pumped to the heat exchanger pressure. Assuming the pump to have an isentropic efficiency of 90 percent, determine:

(a) the isentropic efficiency of the turbine,
(b) the net power output of the plant, and
(c) the thermal efficiency of the cycle.

Answer 1

a. 34.69%

b. 20165.82 kJ/s

c. 12.4

Step-by-step explanation:

Assumptions:

1. Ideal rankine cycle

2. Isentropic stage 3-4

3. Isochoric process - constant volume

4. Isobutane is not a monoatomic gas, therefore cv is used for specific heat

a) The turbine stage is from 3 to 4

We know the inlet and outlet temperatures and pressures of the turbine. We can use butane thermodynamic tables to determine the enthalpies of stage 3 and stage 4

s₄=s₃

h₃=761.54kJ/kg

s₃=2.5457kg/kJ

P₄=410kPa

P₃=3250kPa

If it is isentropic whe can find the isentropic enthalpy by using the the entropy value of s₃ and the P₄:

h₄₍s₎ = 470.40 kJ/kg

Using the T₄:

h₄=689.74kJ/kg

The actual work of the turbine is defined as:

Wₐ = m(h₃-h₄)=m(761.54-689.74)=71.8m

Isentropic work = m (h₃-h₄₍s₎)=m(761.54-470.40)=290.84m

Isentropic work = actual work/isentropic work

`=71.8/290.84=0.2468`

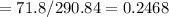
24.68

b)

To work out the total work output we need to determine the work of the pump.

We assume the Pressure is constant through the heat exchanger.

P₁=P₄

h₁ = 273.01 kJ/kg @ 410 kPa

v₁ = 0.001842 m³/kg

Wp,in = mv₁(P₂-P₁)/n

We determine the work of the pump:

Wp,in = 305.6*0.001842*(3250-410)/0.9=1776.26 kJ/s

Wout = Wp,in-Wt = 1776.26-305.6*71.8=20165.82 kJ/s out

c) Thermal efficiency is the Work out divided by the heat put in:

We have the water properties and have h₂ and h₃

Q = 162656 kJ/s

W = 20165.82 kJ/s

n = W/Q = 20165.82/162656=0.1240

Answer 2

A binary geothermal power operates on the simple Rankine cycle with isobutane as the working fluid. The isentropic efficiency of the turbine, the net power output, and the thermal efficiency of the cycle are to be determined

Assumptions :

1. Steady operating conditions exist.

2. Kinetic and potential energy changes are negligible.

Properties: The specific heat of geothermal water (
`c_(geo)`[) is taken to be 4.18 kJ/kg.ºC.

Analysis (a) We need properties of isobutane, we can obtain the properties from EES.

a. Turbine

P
`P_(3) = 3.25mPa = (3.25*1000) kPa\\= 3250kPa\\from the EES TABLE\\h_(3) = 761.54 kJ/kg\\s_(3) = 2.5457 kJ/kg\\P_(4) = 410kPa\\\\s_(4) = s_(3) \\h_(4s) = 470.40kJ/kg\\\\T_(4) = 179.5^(0) C\\\\h_(4) = 689.74 kJ/KG\\\\ The  isentropic  efficiency, n_(T) = (h_(3)-h_(4)  )/(h_(3)- h_(4s) )`

=
`=(761.54-689.74)/(761.54-670.40) \\=(71.8)/(91.14) \\=0.788`

b. Pump

`h_(1) = h_(f) @ 410kPa = 273.01kJ/kg\\v_(1) = v_(f) @ 410kPa = 0.001842 m^(3)/kgw_(p,in) =  (v_(1)(P_(2)-P_(1))   )/(n_(p) ) \\\\= (0.01842(3250-410))/(0.9) \\\\ =5.81kJ/kg\\h_(2) =h_(1) + w_(p,in)\\          = 273.01+5.81\\           = 278.82 kJ/kg\\\\w_(T,out) = m^(.)  (h_(3) -h_(4) )\\=(305.6)(761.54-689.74)\\=305.6(71.8)\\=21,942kW\\\\`

c.
`The thermal efficiency of the cycle  n_(th)  =\frac{W^(.) _(net) }{Q^{._(in) } } \\\\= (20,166)/(162,656) \\=0.124\\=12.4%`