Question

In an experiment, a 0.4351 g sample of benzil (C14H10O2) is burned completely in a bomb calorimeter. The calorimeter is surrounded by 1.153×103 g of water. During the combustion the temperature increases from 25.10 to 27.60 °C. The heat capacity of water is 4.184 J g-1°C-1. The heat capacity of the calorimeter was determined in a previous experiment to be 994.1 J/°C. Assuming that no energy is lost to the surroundings, calculate the molar heat of combustion of benzil based on these data. C14H10O2(s) + (31/2) O2(g) 5 H2O(l) + 14 CO2(g) + Energy Molar Heat of Combustion = kJ/mol g

Answer 1

The enthalpy change during the reaction is -7020.09 kJ/mole.

Step-by-step explanation:

First we have to calculate the heat gained by the calorimeter.

`q=c* (T_(final)-T_(initial))`

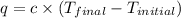
where,

q = heat gained = ?

c = specific heat =
`994.1 J/^oC`

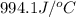
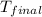`T_(final)` = final temperature =
`27.60^oC`

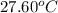
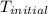`T_(initial)` = initial temperature =
`25.10^oC`

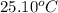
Now put all the given values in the above formula, we get:

`q=994.1 J/^oC* (27.60-25.10)^oC`

`q= 2,485.25 J`

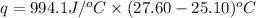
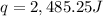
The heat gained by water present in calorimeter. = q'

`q'=mc* (T_(final)-T_(initial))`

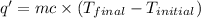
where,

q' = heat gained = ?

m = mass of water =
`1.153* 10^3 g`

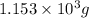
c' = specific heat of water =
`4.184 J/^oC`

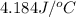
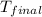`T_(final)` = final temperature =
`27.60^oC`

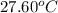
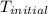`T_(initial)` = initial temperature =
`25.10^oC`

`q'=1.153* 10^3 g* 4.184 J/^oC* (27.60-25.10)^oC`

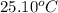
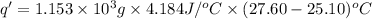
q ' = 12,060.38 J

Now we have to calculate the enthalpy change during the reaction.

`\Delta H=-(Q)/(n)`

where,

`\Delta H` = enthalpy change = ?

Q = heat gained = -(q+q') = -(2,485.25 J + 12,060.38 J)= -14,545.63 J

Q = -14.54563 kJ

n = number of moles fructose =
`\frac{\text{Mass of benzil}}{\text{Molar mass of benzil}}=(0.4351 g)/(210 g/mol)=0.002072 mole`

`\Delta H=-(-14.54563 kJ)/(0.002072 mole)=-7020.09 kJ/mole`

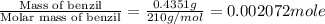
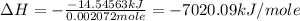
Therefore, the enthalpy change during the reaction is -7020.09 kJ/mole.