Question

A Carnot heat engine uses a hot reservoir consisting of a large amount of boiling water and a cold reservoir consisting of a large tub of ice and water. In 5 minutes of operation, the heat rejected by the engine melts 0.0400 kg of ice. During this time, how much work W is performed by the engine?

Answer 1

W = 4893.77 J

Step-by-step explanation:

given,

mass of the ice = 0.04 Kg

time of operation = 5 minutes

Heat delivered =

`Q_c = m_c \Delta H_f`

Δ H_f is latent heat of fusion of ice = 334 x 10³ J/Kg

`Q_c = 0.04 * 334 * 10^3`

`Q_c = 13360\ J`

Work done

W = Q_H - Q_C

`Q_H= ((T_H)/(T_C))Q_C`

`W= ((T_H)/(T_C)-1)Q_C`

`W= ((373)/(273)-1)* 13360`

W = 4893.77 J

Answer 2

W= 4.89 KJ

Step-by-step explanation:

Lets take

temperature of hot water T₁ = 100⁰C

T₁ = 373 K

Temperature of cold ice T₂= 0⁰C

T₂ = 273 K

The latent heat of ice LH= 334 KJ

The heat rejected by the engine Q= m .LH

Q₂= 0.04 x 334

Q₂= 13.36 KJ

Heat gain by engine = Q₁

For Carnot engine

`Q_1=(T_1)/(T_2)Q_2`

`Q_1=(373)/(273)* 13.36`

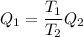
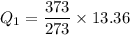
Q₁ = 18.25 KJ

The work W= Q₁ - Q₂

W= 18.25 - 13.36 KJ

W= 4.89 KJ