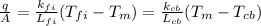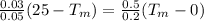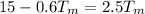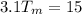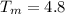Question

A composite wall is composed of 20 cm of concrete block with k = 0.5 W/m-K and 5 cm of foam insulation with k = 0.03 W/m-K. The temperature of on the foam side is 25oC while the temperature on the block side is 0oC. What is the temperature at the interface between the block and the foam?

Answer 1

4.8°C

Step-by-step explanation:

The rate of heat transfer through the wall is given by:

`q=(Ak)/(L)dT`

`(q)/(A)=(k)/(L)dT`

Assumptions:

1) the system is at equilibrium

2) the heat transfer from foam side to interface and interface to block side is equal. There is no heat retention at any point

3) the external surface of the wall (concrete block side) is large enough that all heat is dissipated and there is no increase in temperature of the air on that side

`{k_(fi)= 0.03 W/m.K`

`{L_(fi)= 5 cm = 0.05 m`

`{T_(fi)= 25 \°C`

`{k_(cb) = 0.5 W/m.K`

`{L_(cb)= 20 cm = 0.20 m`

`{T_(cb)= 0 \°C`

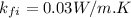
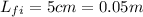
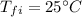
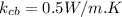
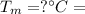`{T_(m)= ? \°C =` temperature at the interface

Solving for
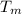`{T_(m)` will give the temperature at the interface:

`(q)/(A)=(k_(fi) )/(L_(fi) )(T_(fi) -T_(m))=(k_(cb) )/(L_(cb) )(T_(m) -T_(cb))`

`(0.03)/(0.05 )(25 -T_(m))=(0.5)/(0.2)(T_(m) -0})`

`15 -0.6T_(m)=2.5T_(m)`

`3.1T_(m)=15`

`T_(m)=4.8`