Question

Find the maximum and minimum values of the given quadratic form subject to the constraint x2 + y2 + z2 = 1 and determine the values of x, y, and z at which the maximum and minimum occur. 5. 9x2 + 4y2 + 3z2

Answer 1

• maximum: 9 at (x, y, z) = (1, 0, 0)
• minimum: 3 at (x, y, z) = (0, 0, 1)

Explanation:

The method of Lagrange multipliers can be used to find the extrema subject to the constraint. The Lagrangian can be written ...

`L=9x^2+4y^2+3z^2+\lambda(x^2+y^2+z^2-1)`

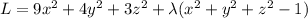
We want to find the solution to the simultaneous equations when the partial derivatives are all zero.

`\displaystyle\left\{\begin{array}{l}(\partial L)/(\partial x)=0=18x+2\lambda x\\\\(\partial L)/(\partial y)=0=8y+2\lambda y \\\\(\partial L)/(\partial z)=0=6z+2\lambda z\\\\(\partial L)/(\partial\lambda)=0=x^2+y^2+z^2-1\end{array}\right.`

These can be simplified to ...

`\displaystyle\left\{\begin{array}{l}0=x(9+\lambda)\\\\0=y(4+\lambda)\\\\0=z(3+\lambda)\\\\0=x^2+y^2+z^2-1\end{array}\right.`

The first of these has solutions x=0 or λ=-9. In the latter case, the other equations require y=z=0 and x=1.

The second has solutions y=0 or λ=-4. In the latter case, the other equations require x=z=0 and y=1.

The third has solutions z=0 or λ=-3. In the latter case, the other equations require x=y=0 and z=1.

The objective function (given quadratic form) has these values at the points just found:

9 for (x, y, z) = (1, 0, 0) . . . . . a maximum

4 for (x, y, z) = (0, 1, 0)

3 for (x, y, z) = (0, 0, 1) . . . . . a minimum

_____

Alternate approach

You can solve the constraint for z^2 and substitute that into the objective function f(x, y, z). It will then be ...

f(x, y) = 9x^2 +4y^2 +3(1 -x^2 -y^2) = 6x^2 +3y^2 +3

Since x^2 and y^2 must be non-negative, the minimum value of this function is clearly 3.

Similarly, you can solve the constraint for x^2 and substitute that into f(x, y, z) to get ...

f(y, z) = 9(1 -y^2 -z^2) +4y^2 +3z^2 = -5y^2 -6z^2 +9

Again, the fact that y^2 and z^2 are zero at least means the maximum value of f(y, z) is 9.