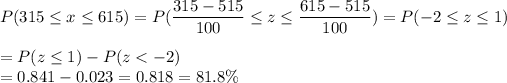Question

The national average for the math portion of the College Board’s Scholastic Aptitude Test

(SAT) is 515 (The World Almanac, 2009). The College Board periodically rescales the test
scores such that the standard deviation is approximately 100. Answer the following questions
using a bell-shaped distribution and the empirical rule for the verbal test scores.

a. What percentage of students have an SAT verbal score greater than 615?
b. What percentage of students have an SAT verbal score greater than 715?
c. What percentage of students have an SAT verbal score between 415 and 515?
d. What percentage of students have an SAT verbal score between 315 and 615?

Answer 1

a) 0.1587

b) 0.023

c) 0.341

d) 0.818

Explanation:

We are given the following information in the question:

Mean, μ = 515

Standard Deviation, σ = 100

We are given that the distribution of SAT score is a bell shaped distribution that is a normal distribution.

Formula:

`z_(score) = \displaystyle(x-\mu)/(\sigma)`

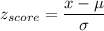
a) P(score greater than 615)

P(x > 615)

`P( x > 615) = P( z > \displaystyle(615 - 515)/(100)) = P(z > 1)`

`= 1 - P(z \leq 1)`

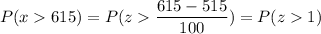
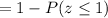
Calculation the value from standard normal z table, we have,

`P(x > 615) = 1 - 0.8413 = 0.1587 = 15.87\%`

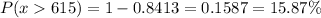
b) b) P(score greater than 715)

`P(x > 715) = P(z > \displaystyle(715-515)/(100)) = P(z > 2)\\\\P( z > 2) = 1 - P(z \leq 2)`

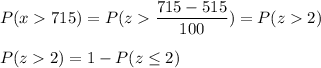
Calculating the value from the standard normal table we have,

`1 - 0.977 = 0.023 = 2.3\%\\P( x > 715) = 2.3\%`

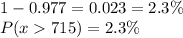
c) P(score between 415 and 515)

`P(415 \leq x \leq 515) = P(\displaystyle(415 - 515)/(100) \leq z \leq \displaystyle(515-515)/(100)) = P(-1 \leq z \leq 0)\\\\= P(z \leq 0) - P(z < -1)\\= 0.500 - 0.159 = 0.341 = 34.1\%`

`P(415 \leq x \leq 515) = 34.1\%`

d) P(score between 315 and 615)

`P(315 \leq x \leq 615) = P(\displaystyle(315 - 515)/(100) \leq z \leq \displaystyle(615-515)/(100)) = P(-2 \leq z \leq 1)\\\\= P(z \leq 1) - P(z < -2)\\= 0.841 - 0.023 = 0.818 = 81.8\%`

`P(315 \leq x \leq 615) = 81.8\%`