Question

Suppose you have two​ populations: Population Along dashAll students at a state college ​(Nequals​21,000) and Population Blong dashAll residents of a town in the state ​(Nequals​21,000). You want to estimate the mean age of each population using two separate samples each of size nequals75. If you construct a​ 95% confidence interval for each population​ mean, will the margin of error for population A be​ larger, the​ same, or smaller than the margin of error for population​ B? Justify your reasoning.

Answer 1

See the explantion below

Explanation:

The margin of error is the range of values below and above the sample statistic in a confidence interval.

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

Assuming the X follows a normal distribution

`X \sim N(\mu, \sigma)`

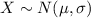
We know that the margin of error for a confidence interval is given by:

`Me=z_(\alpha/2)(\sigma)/(√(n))` (1)

If we see the formula (1) at the same confidence level, for example 95% and with the same sample size the margin of error just depends of the deviation. If the population deviation for population A is higher than the population deviation for B then A will have more margin of error than B.

On the other case if the deviation for population A is lower than the deviation for population B, then we will have less margin of error for population A than population B.

And the other possible case if both population have the same deviation, then both have equal margin of error.