Question

A certain substance X has a normal freezing point of -6.4 C and a molal freezing point depression constant Kf= 3.96 degrees C.kg.mol-1. A solution is prepared by dissolving some urea ((NH2)2CO) in 950. g of X. This solution freezes at -13.6 C. calculate the mass of urea that was dissolved. Round your answer to 2 significant digits.

Answer 1

Answer:
`1.0* 10^2g`

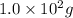
Step-by-step explanation:

Depression in freezing point is given by:

`\Delta T_f=i* K_f* m`

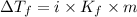
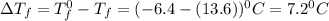`\Delta T_f=T_f^0-T_f=(-6.4-(13.6))^0C=7.2^0C` = Depression in freezing point

i= vant hoff factor = 1 (for non electrolyte like urea)

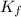`K_f` = freezing point constant =
`3.96^0C/m`

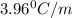
m= molality

`\Delta T_f=i* K_f* \frac{\text{mass of solute}}{\text{molar mass of solute}}* \text{weight of solvent in kg}}`

Weight of solvent (X)= 950 g = 0.95 kg

Molar mass of non electrolyte (urea) = 60.06 g/mol

Mass of non electrolyte (urea) added = ?

`7.2=1* 3.96* (xg)/(60.06 g/mol* 0.95kg)`

`x=1.0* 10^2g`

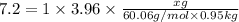
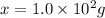
Thus
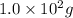`1.0* 10^2g` urea was dissolved.