Question

Suppose a disk rotates at constant angular velocity.

(a) Does a point on the rim have radial and or tangential acceleration?
(b) If the disk’s angular velocity increases uniformly, does the point have radial and or tangential acceleration?
(c) For which cases would the magnitude of either component of linear acceleration change

Answer 1

Step-by-step explanation:

(a)Disc rotates at constant angular velocity

constant angular velocity denotes zero angular acceleration therefore disc has only radial acceleration and no tangential acceleration

because
`a_r=\omega * r`

`a_t=(dv)/(dt)=0`

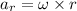
(b)If disk angular velocity is increasing uniformly it implies there is presence of constant angular acceleration

therefore Point on disc have both tangential and radial acceleration

(c)For First case

Neither component of linear acceleration is changing as disc rotates with constant angular velocity

For second case only radial component of linear acceleration is changing while tangential acceleration is same.