Step-by-step explanation:
It is given that,
Frequency of the laser light,
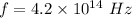
Time,
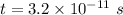
(a) Let
 is the wavelength of this light. It can be calculated as :
is the wavelength of this light. It can be calculated as :
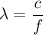
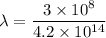
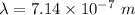
or
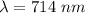
(b) Let n is the number of the wavelengths in one pulse. It can be calculated as :
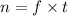

n = 13440
Hence, this is the required solution.